Thermal Blooming: Difference between revisions
No edit summary |
No edit summary |
||
| (14 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
A high powered laser going through the air will cause the air to heat up. If the laser beam lasts longer than a small fraction of a second, the hot air will expand and become less dense. Less dense air has a lower index of refraction, so the density gradient across the beam acts like a lens | A high powered laser going through the air will cause the air to heat up due to [[Attenuation#Absorption|absorption]]. If the laser beam lasts longer than a small fraction of a second, the hot air will expand and become less dense. Less dense air has a lower index of refraction, so the density gradient across the beam acts like a lens which in this case makes the beam expand. This expansion is called <i>thermal blooming</i>. The phenomenon is non-linear - if you try to compensate for not enough intensity on target by increasing the beam power without also making the beam wider to compensate, you can just end up heating the air more and actually reducing the beam intensity. | ||
[[File:Thermal_blooming_in_wind.png|thumb|Bending of the beam from an unperturbed circular shape (dashed circle) into a crescent shape due to thermal blooming in wind<ref name=Gebhardt_1990>Frederick Gebhardt, “Twenty-five years of thermal blooming: an overview”, Proc. SPIE 1221, Propagation of High-Energy Laser Beams Through the Earth’s Atmosphere, (1 May 1990); doi: 10.1117/12.18326</ref>]] | [[File:Thermal_blooming_in_wind.png|thumb|Bending of the beam from an unperturbed circular shape (dashed circle) into a crescent shape due to thermal blooming in wind<ref name=Gebhardt_1990>Frederick Gebhardt, “Twenty-five years of thermal blooming: an overview”, Proc. SPIE 1221, Propagation of High-Energy Laser Beams Through the Earth’s Atmosphere, (1 May 1990); doi: 10.1117/12.18326</ref>]] | ||
| Line 6: | Line 6: | ||
Adaptive optics methods can help to solve this, but would-be laser engineers need to be careful because a straightforward implementation just ends up making the problem worse (to counteract the expansion, you focus the beam more which heats up the air more which makes your beam diverge even more). Alternatively, you can use high power laser pulses that are so short that the air does not have time to expand. | Adaptive optics methods can help to solve this, but would-be laser engineers need to be careful because a straightforward implementation just ends up making the problem worse (to counteract the expansion, you focus the beam more which heats up the air more which makes your beam diverge even more). Alternatively, you can use high power laser pulses that are so short that the air does not have time to expand. | ||
Does your beam need to worry about thermal blooming? For relatively long duration beams moving relative to the air you can compute the <i>thermal distortion factor</i>, | Does your beam need to worry about thermal blooming? For relatively long duration beams moving relative to the air you can compute the <i>thermal distortion factor</i>, N, to find out. If N is less than one, you can ignore thermal blooming. If N is greater than this, you will need to correct for blooming if you want to keep a tight spot size. The larger N, the more difficult the blooming will be to correct. | ||
To compute | To compute N | ||
<ref name=Gebhardt_1990>Frederick Gebhardt, “Twenty-five years of thermal blooming: an overview”, Proc. SPIE 1221, Propagation of High-Energy Laser Beams Through the Earth’s Atmosphere, (1 May 1990); doi: 10.1117/12.18326</ref> | <ref name=Gebhardt_1990>Frederick Gebhardt, “Twenty-five years of thermal blooming: an overview”, Proc. SPIE 1221, Propagation of High-Energy Laser Beams Through the Earth’s Atmosphere, (1 May 1990); doi: 10.1117/12.18326</ref> | ||
(Warning! Math ahead!) | (Warning! Math ahead!) | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;">< | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
<table> | |||
<tr><td>N<sub>c</sub> <td> = <td align="left"> [16 √2 / π] [β P R² / (R<sub>a</sub> v D³] | |||
<tr><td>N<sub>E</sub> <td> = <td align="left"> R / R<sub>0</sub> | |||
f | <tr><td rowspan=2> f <td rowspan=2> = <td align="left">1 if N<sub>E</sub> = 0 | ||
<tr> <td align="left">(2 / N<sub>E</sub>²) [N<sub>E</sub> - 1 + exp(-N<sub>E</sub>)] otherwise | |||
<tr><td> N<sub>F</sub> <td> = <td align="left"> (π/4) n D / S | |||
<tr><td rowspan=2> q <td rowspan=2> = <td align="left">1 if N<sub>F</sub> = 1 | |||
<tr> <td align="left">[(2 N<sub>F</sub>²) / (N<sub>F</sub> - 1)] [1 - ln(N<sub>F</sub>)/(N<sub>F</sub> - 1)] otherwise | |||
<tr><td> N<sub>V</sub> <td> = <td align="left"> v<sub>T</sub> / v | |||
<tr><td rowspan=2> s <td rowspan=2> = <td align="left">1 if N<sub>V</sub> = 1 | |||
q | <tr> <td align="left">[2 / (N<sub>V</sub> - 1)²] [N<sub>V</sub> ln(N<sub>V</sub>) - (N<sub>V</sub> - 1)] otherwise | ||
<tr><td> N <td> = <td align="left"> N<sub>c</sub> f q s | |||
</table> | |||
</div> | |||
where S is the beam spot size diameter at the target, D is the beam diameter at the aperture, and R is the range to the target. P is the beam power. The relative wind speed to the beam is v at the aperture and v<sub>T</sub> at the target, and n is the refractive index of the air. R<sub>a</sub> is the [[Attenuation#The_Beer-Lambert_law|characteristic absorption length]] of the wavelength of light you are using for the given atmospheric conditions, and R<sub>0</sub> is the net [[Attenuation#The_Beer-Lambert_law|attenuation length]] including both absorption and scattering. Remember that R<sub>a</sub> is the absorption length, not the total attenuation length, so primarily scattering phenomena like clouds or fog or mist won’t change it much. | |||
s | |||
N | |||
</ | |||
where | |||
The parameter | The parameter β is the atmospheric thermal coefficient | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;" | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
β = (-dn/dT) / (ρ C<sub>P</sub>) | |||
</ | </div> | ||
where | where dn/dT is the rate of change of the refractive index with increasing temperature, ρ is the air’s mass density, C<sub>P</sub> is the specific heat capacity of the air at constant pressure, and β in sea level air on earth under typical conditions at visible and near visible wavelengths is around β = 8.3 × 10<sup>-10</sup> m³/J. | ||
If there is no wind speed, you can take the speed at which the heated air rises out of the beam path (a process known as convection) instead. | If there is no wind speed, you can take the speed at which the heated air rises out of the beam path (a process known as convection) instead. | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;">< | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
v<sub>convection</sub> = ( (2 P g) / (ρ C<sub>P</sub> R<sub>a</sub> T) )<sup>1/3</sup> | |||
</ | </div> | ||
where | where g is the acceleration due to gravity (g = 9.8 m/s² on Earth). Normally, however, if you are outside this will be small compared to any natural wind in the environment. And if you are inside the range is probably too short to matter. | ||
For pulsed beams, thermal blooming can be less of an issue. For one thing, the pulse is usually so fast that wind has a negligible effect. If your pulse length is shorter than your minimum beam diameter divided by the speed of sound, thermal blooming is much less of an issue because the air will not have had enough time to expand to make a lens. The equivalent of the thermal distortion factor | For pulsed beams, thermal blooming can be less of an issue. For one thing, the pulse is usually so fast that wind has a negligible effect. If your pulse length is shorter than your minimum beam diameter divided by the speed of sound, thermal blooming is much less of an issue because the air will not have had enough time to expand to make a lens. The equivalent of the thermal distortion factor N for pulsed beams are the parameters N<sub>l</sub> and N<sub>s</sub> for pulses long and short compared to the time for sound to cross their beam diameters, respectively. | ||
<ref name=Kleiman_1973>H. Kleiman and R. W. O'Neil "Thermal blooming of pulsed laser radiation", Appl. Phys. Lett. 23, 43 (1973). < | <ref name=Kleiman_1973>H. Kleiman and R. W. O'Neil "Thermal blooming of pulsed laser radiation", Appl. Phys. Lett. 23, 43 (1973). N<sub>l</sub> differs by a factor of 2 from T<sub>lc</sub> and N<sub>s</sub> by a factor of 4 from T<sub>sc</sub> because those give the time-dependent change in the laser spot and we are interested in the total fluence dumped on the target during the time the spot is on. So the presented results are for time-averaging the T parameters.</ref> | ||
<div class="center" style="width: auto; margin-left: auto; margin-right: auto;">< | <div class="center" style="width: auto; margin-left: auto; margin-right: auto;"> | ||
<table> | |||
<tr><td>N<sub>l</sub> <td> = <td align="left"> (1 / (6 π)) [(β E R<sup>2</sup>) / (R<sub>a</sub> D<sup>2</sup> S<sup>2</sup>)] | |||
<tr><td>N<sub>s</sub> <td> = <td align="left"> (1 / (30 π)) [(β E R<sup>2</sup> (t c<sub>s</sub>)<sup>2</sup>) / (R<sub>a</sub> D<sup>2</sup> S<sup>4</sup>)] | |||
</table> | |||
</ | </div> | ||
where | where E is the pulse energy, t is the pulse duration, c<sub>s</sub> is the speed of sound (approximately 330 m/s in sea level air at shirt-sleeve temperatures). If D / c<sub>s</sub> < t, use N<sub>l</sub>. If S / c<sub>s</sub> > t, use N<sub>s</sub>. If you just happen to be somewhere between these, either take your best guess or get a grant, hire several grad students and post-docs, and perform the research to figure out the proper formula yourself. Again, if N<sub>l</sub> or N<sub>s</sub>, as appropriate, is less than one thermal blooming is not much of an issue. If they are greater than one, either use sophisticated non-linear adaptive optics beam controls or adjust your beam parameters so it is less than one. | ||
It is interesting to note that for [[Diffraction|diffraction]]-limited beams, R / (S D) does not depend on distance so long as the beam is focused at the target. So a pulsed beam governed by N<sub>l</sub> has the same amount of thermal blooming at any distance. | |||
==Credit== | |||
Author: Luke Campbell | |||
==References== | |||
[[Category:Lasers]][[Category:Physics]][[Category:Heat]][[Category:Physics & Engineering]] | |||
Latest revision as of 18:19, 9 April 2025
A high powered laser going through the air will cause the air to heat up due to absorption. If the laser beam lasts longer than a small fraction of a second, the hot air will expand and become less dense. Less dense air has a lower index of refraction, so the density gradient across the beam acts like a lens which in this case makes the beam expand. This expansion is called thermal blooming. The phenomenon is non-linear - if you try to compensate for not enough intensity on target by increasing the beam power without also making the beam wider to compensate, you can just end up heating the air more and actually reducing the beam intensity.
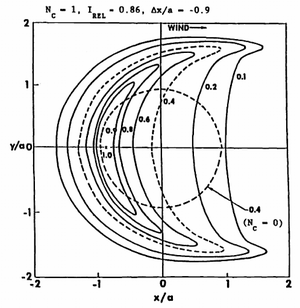
If there is wind, or if you are moving quickly through the air (like if you are flying an airplane), or your target is (like if you are shooting at missiles or artillery shells), you are continually getting fresh air into your beam. This makes the air that has been in your beam for longer hotter than the newer air, forming a lens which bends your beam in addition to expanding it.
Adaptive optics methods can help to solve this, but would-be laser engineers need to be careful because a straightforward implementation just ends up making the problem worse (to counteract the expansion, you focus the beam more which heats up the air more which makes your beam diverge even more). Alternatively, you can use high power laser pulses that are so short that the air does not have time to expand.
Does your beam need to worry about thermal blooming? For relatively long duration beams moving relative to the air you can compute the thermal distortion factor, N, to find out. If N is less than one, you can ignore thermal blooming. If N is greater than this, you will need to correct for blooming if you want to keep a tight spot size. The larger N, the more difficult the blooming will be to correct.
To compute N [1] (Warning! Math ahead!)
| Nc | = | [16 √2 / π] [β P R² / (Ra v D³] |
| NE | = | R / R0 |
| f | = | 1 if NE = 0 |
| (2 / NE²) [NE - 1 + exp(-NE)] otherwise | ||
| NF | = | (π/4) n D / S |
| q | = | 1 if NF = 1 |
| [(2 NF²) / (NF - 1)] [1 - ln(NF)/(NF - 1)] otherwise | ||
| NV | = | vT / v |
| s | = | 1 if NV = 1 |
| [2 / (NV - 1)²] [NV ln(NV) - (NV - 1)] otherwise | ||
| N | = | Nc f q s |
where S is the beam spot size diameter at the target, D is the beam diameter at the aperture, and R is the range to the target. P is the beam power. The relative wind speed to the beam is v at the aperture and vT at the target, and n is the refractive index of the air. Ra is the characteristic absorption length of the wavelength of light you are using for the given atmospheric conditions, and R0 is the net attenuation length including both absorption and scattering. Remember that Ra is the absorption length, not the total attenuation length, so primarily scattering phenomena like clouds or fog or mist won’t change it much.
The parameter β is the atmospheric thermal coefficient
β = (-dn/dT) / (ρ CP)
where dn/dT is the rate of change of the refractive index with increasing temperature, ρ is the air’s mass density, CP is the specific heat capacity of the air at constant pressure, and β in sea level air on earth under typical conditions at visible and near visible wavelengths is around β = 8.3 × 10-10 m³/J.
If there is no wind speed, you can take the speed at which the heated air rises out of the beam path (a process known as convection) instead.
vconvection = ( (2 P g) / (ρ CP Ra T) )1/3
where g is the acceleration due to gravity (g = 9.8 m/s² on Earth). Normally, however, if you are outside this will be small compared to any natural wind in the environment. And if you are inside the range is probably too short to matter.
For pulsed beams, thermal blooming can be less of an issue. For one thing, the pulse is usually so fast that wind has a negligible effect. If your pulse length is shorter than your minimum beam diameter divided by the speed of sound, thermal blooming is much less of an issue because the air will not have had enough time to expand to make a lens. The equivalent of the thermal distortion factor N for pulsed beams are the parameters Nl and Ns for pulses long and short compared to the time for sound to cross their beam diameters, respectively. [2]
| Nl | = | (1 / (6 π)) [(β E R2) / (Ra D2 S2)] |
| Ns | = | (1 / (30 π)) [(β E R2 (t cs)2) / (Ra D2 S4)] |
where E is the pulse energy, t is the pulse duration, cs is the speed of sound (approximately 330 m/s in sea level air at shirt-sleeve temperatures). If D / cs < t, use Nl. If S / cs > t, use Ns. If you just happen to be somewhere between these, either take your best guess or get a grant, hire several grad students and post-docs, and perform the research to figure out the proper formula yourself. Again, if Nl or Ns, as appropriate, is less than one thermal blooming is not much of an issue. If they are greater than one, either use sophisticated non-linear adaptive optics beam controls or adjust your beam parameters so it is less than one.
It is interesting to note that for diffraction-limited beams, R / (S D) does not depend on distance so long as the beam is focused at the target. So a pulsed beam governed by Nl has the same amount of thermal blooming at any distance.
Credit
Author: Luke Campbell
References
- ↑ Jump up to: 1.0 1.1 Frederick Gebhardt, “Twenty-five years of thermal blooming: an overview”, Proc. SPIE 1221, Propagation of High-Energy Laser Beams Through the Earth’s Atmosphere, (1 May 1990); doi: 10.1117/12.18326
- ↑ H. Kleiman and R. W. O'Neil "Thermal blooming of pulsed laser radiation", Appl. Phys. Lett. 23, 43 (1973). Nl differs by a factor of 2 from Tlc and Ns by a factor of 4 from Tsc because those give the time-dependent change in the laser spot and we are interested in the total fluence dumped on the target during the time the spot is on. So the presented results are for time-averaging the T parameters.